Given
A bag contains 13 red balls numbered 1-13 and 5 green balls numbered 14-18.
And a ball is chosen at random.
To find:
a) The probability that you choose a ren or even numbered ball.
b) The the probability you choose a green ball or a ball numbered less than 5.
Step-by-step explanation:
It is given that,
The total number of balls is (13+5)=18.
The number of red balls is 13 numbered from 1-13.
The number of green balls is 5 numbered from 14-18.
i) Then, the probability of getting a a red or even numbered ball is,
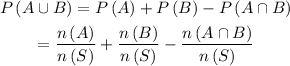
Here,
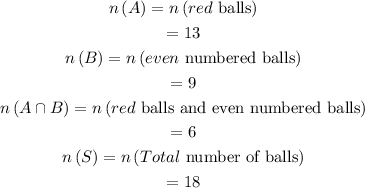
That implies,
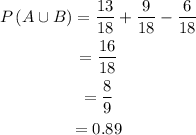
Hence, the probability of getting a red or even numbered ball is 0.89.
ii) Also,
The probability of getting a green ball or a ball numbered less than 5 is,
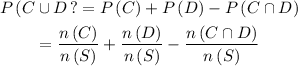
Here,
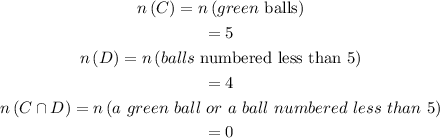
Then,

Hence, the probabilty of getting a green ball or a ball numbered less than 5 is 0.11.