Question

Consider the first few terms as well as the last few terms of the sum. Find a way to simplify and use your observation to evaluate the sum. Write the exact answer. Do not round.

Answer 1

The first three terms of the sum are:

`\begin{gathered} (1)/(3)-(1)/(3+1)=(1)/(3)-(1)/(4)\to\text{first} \\ (1)/(4)-(1)/(4+1)=(1)/(4)-(1)/(5)\to\text{second} \\ (1)/(5)-(1)/(6)\to\text{ third} \end{gathered}`

On the other hand, the three last terms of the sum are

`\begin{gathered} (1)/(10)-(1)/(10+1)=(1)/(10)-(1)/(11)\to\text{tenth} \\ (1)/(11)-(1)/(12)\to\text{ eleventh} \\ (1)/(12)-(1)/(13)\to\text{twelfth} \end{gathered}`

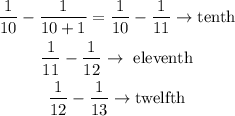
Notice that there is a -1/4 in the first term, a 1/4 in the second term, a 1/5 in the second term, and 1/5 in the third term, and so on. Once we add those factors, the result will be zero. Thus, the result of the sum is equal to the first number in the first term minus the second number in the twelfth term.

`\begin{gathered} \sum ^(12)_(i=3)((1)/(i)-(1)/(i+1))=(1)/(3)-(1)/(13)=(13-3)/(39)=(10)/(39) \\ \Rightarrow\sum ^(12)_(i=3)((1)/(i)-(1)/(i+1))=(10)/(39) \end{gathered}`

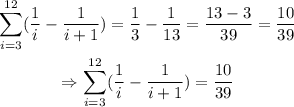
The answer is 10/39