Question

I would like to know if I answered the question correctly

Answer 1

INFORMATION:

We have the next system of equations:

`\begin{cases}{x-5y=-16} \\ {9x+9y=72} \\ {4x-6z=-8}\end{cases}`

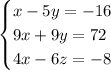
And we need to determine if (4, 4, 4) is a solution of the system.

STEP BY STEP EXPLANATION:

To know if the ordered triple is a solution of the system, we need to that (4, 4, 4) means x = 4, y = 4 and z = 4.

Then, to know if it is a solution we must replace the values on each equation to verify if the values are solutions

We have three equations:

1. x - 5y = -16

Replacing x = 4 and y = 4, we obtain

`\begin{gathered} 4-5\cdot4=-16 \\ 4-20=-16 \\ -16=-16 \\ \text{ TRUE} \end{gathered}`

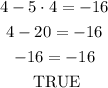
2. 9x + 9y = 72

Replacing x = 4 and y = 4, we obtain

`\begin{gathered} 9\cdot4+9\cdot4=72 \\ 36+36=72 \\ 72=72 \\ \text{ TRUE} \end{gathered}`

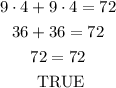
3. 4x - 6z = -8

Replacing x = 4 and z = 4, we obtain

`\begin{gathered} 4\cdot4-6\cdot4=-8 \\ 16-24=-8 \\ -8=-8 \\ \text{ TRUE} \end{gathered}`

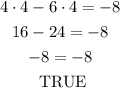
Finally, since the three equations are true when x = 4, y = 4 and z = 4, the ordered triple is a solution

ANSWER:

Yes