Question

Find the slope of the tangent line to f(x) when x= -3. Two points on the line tangent to f(x) at x = -3 are: (-4,-7) and (1,3).

Answer 1

When x = -3, the line that's tangent to f(x) is shown in the given image. So, in order to find the slope of f(x) at x = -3, we need to find the slope of that line.

The slope of a line is given by the formula:

`(y_2-y_1)/(x_2-x_1)`

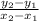
Now, notice that the points (-4,-7) and (1,3) belong to the tangent line. Therefore, we can use them to find the slope:

y₂ = 3

y₁ = -7

x₂ = 1

x₁ = -4

So, we have:

`(y_2-y_1)/(x_2-x_1)=(3-(-7))/(1-(-4))=(3+7)/(1+4)=(10)/(5)=2`

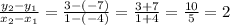
Therefore, the slope is 2.