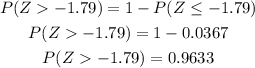Question

For a standard normal distribution, find:P(Z > -1.79)Express the probability as a decimal rounded to 4 decimalplaces.

Answer 1

Given the probability:

`P(Z>-1.79)`

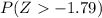
To find this probability, you have to use the tables for the standard normal distribution. These tables show the accumulated probability for the Z-distribution, this means that you can find the probability up to a determined Z-value. To find the probability above a determined Z-value you have to use the complementary probability, that is:

`P(Z>-1.79)=1-P(Z\leq-1.79)`

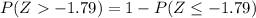
- First, use the Z-table to find the accumulated probability until Z=-1.79, the Z-value is negative, so you have to use the left entry of the table:

In the first column, you will find the first two digits of the Z-value, and in the first two, you will find the third digit of the Z-value, and in the body of the table, all accumulated probabilities are listed.

Cross the corresponding row and column to find the accumulated probability:

Then the accumulated probability until -1.79 is:

`P(Z\leq-1.79)=0.0367`

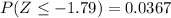
Now that you found the accumulated probability, you can use the complement to find the probability above -1.79

`\begin{gathered} P(Z>-1.79)=1-P(Z\leq-1.79) \\ P(Z>-1.79)=1-0.0367 \\ P(Z>-1.79)=0.9633 \end{gathered}`