Answer
The mass of oxygen needed to burn 54.0g of butane is 193.24 g
Step-by-step explanation
Given:
Mass of butane = 54.0 g
What to find:
The mass of oxygen needed to burn 54.0g of butane.
Step-by-step solution:
Step 1: Write the balanced chemical equation for the reaction.
2C₄H₁₀ + 13O₂ → 8CO₂ + 10H₂O
Step 2: Convert 54.0 g of butane to moles
Molar mass of C₄H₁₀ = 58.12 g/mol
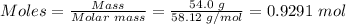
Step 3: Use the mole ratio in step one and the mole of C₄H₁₀ in step 2 to calculate the mole of oxygen.
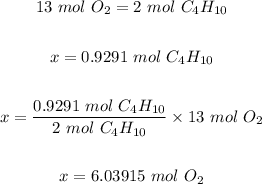
Step 4: Convert the moles of O₂ in step 3 to mass in grams.
Molar mass of O₂ = 31.998 g/mol
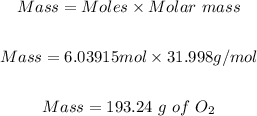
Hence, the mass of oxygen needed to burn 54.0g of butane is 193.24 g