Given the equation of the elliptical orbit is (x+2.5)^2/22,350.25+y^2/22,344=1.
This equation can be written as
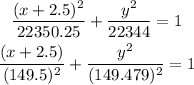
Now, if we shift this path by 2.5 units to left then we get
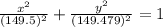
The farthest distance of the earth from the sun will be 149.5 - 2.5 = 147 million of kilometers
Thus, option C is correct.