From the question, we can deduce the answer using indices which deals with power of numbers, e.g

Also, for the next one,
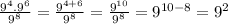
This applies to every other ones provided in the question,
Considering Option D,

Since the equation D favours the solution,
Hence, the best option is D.