The given triangle is right angle triangle with side a = 8 and b 7
Apply pythagoras theorem for the side c;
In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides.
Hypotenuse² = Perpendicular² + Base²
Here, perpendicular, a =8 and Base b = 7
![\begin{gathered} c^(2)=a^(2)+b^(2) \\ c^(2)=8^(2)+7^(2) \\ c^2=113 \\ c=\sqrt[]{113} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/oyu11jjoy0rshvm43vkjteo9up49eomael.png)
The trignometric ratio for sec of an angle define as the ratio of the hypotenuse to the side adjacent to a given angle in a right triangle.
![\begin{gathered} \sec A=\frac{Hypotenuse}{Adjacent\text{ side of angle A}} \\ \sec A=(c)/(b) \\ \sec A=\frac{\sqrt[]{113}}{7} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/u9mieoxttj25oqhsh6assshpcbn49q80m9.png)
The trignometric ratio for cosine of angle define as the ratio of the adjacent side to the the opposite side of the angle,
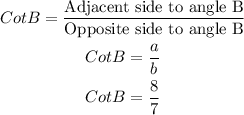
Answer; a)
![\text{SecA}=\frac{\sqrt[]{113}}{7},\cot B=(8)/(7)](https://img.qammunity.org/2023/formulas/mathematics/college/tslq8m9axi161ee2wwa4sfbhajq1q226p4.png)