Solution
Given that
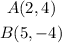
To find the slope, m, of the line passing through the given points, the formula is

Where
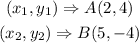
Substitute the coordinates into the formula to find the slope, m, of a line
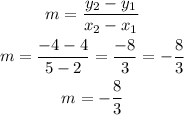
The slope of the line AB passing through the given points is m = -8/3
A) If two lines are parallel, their slopes are equal.
Hence, the slope, m₁ of the line that is parellel to line AB is
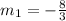
Thus, the slope of a line parallel to line AB is m₁ = -8/3
B) If two lines are perpendicular, the formula to find the slope m₂ of the line perpedicular to the slope of a given line
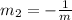
Where m = -8/3, the slope, m₂, of a line perpendicular to line AB will be
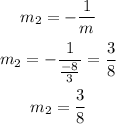
Thus, the slope of a line perpendicular to line AB is m₂ = 3/8