Solve the following system of equations and state whether the system is dependent, independent, or inconsistent.4x+3y=12And4x-3y=12

Question

asked Aug 11, 2023 173k views

1 Answer

Calvin Nunes · Answer 1 · 2023-08-16T23:24:24+0000

Given:

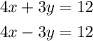
$\begin{gathered} 4x+3y=12 \\ 4x-3y=12 \end{gathered}$

Required:

To solve the system of equation using graph and to state whether the system is dependent, independent, or inconsistent.

Step-by-step explanation:

Consider the equation

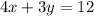
When x=0,

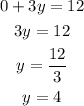
$\begin{gathered} 0+3y=12 \\ 3y=12 \\ y=(12)/(3) \\ y=4 \end{gathered}$

When x=3,

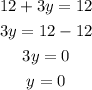
$\begin{gathered} 12+3y=12 \\ 3y=12-12 \\ 3y=0 \\ y=0 \end{gathered}$

Now consider the equation

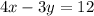
When x=0,

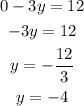
$\begin{gathered} 0-3y=12 \\ -3y=12 \\ y=-(12)/(3) \\ y=-4 \end{gathered}$

When x= 3,

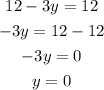
$\begin{gathered} 12-3y=12 \\ -3y=12-12 \\ -3y=0 \\ y=0 \end{gathered}$

The graph of the given system of equation is,

The blue graph is graph of 4x+3y=12 and the black graph is graph of

4x-3y=12.

The two line crosses at the point (3,0).

Therefore the solution is

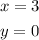
$\begin{gathered} x=3 \\ y=0 \end{gathered}$

Here the solution is one.

Therefore the consistent system has exactly one solution, it is independent .

Final Answer:

The solution of the given system of equation is

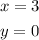
$\begin{gathered} x=3 \\ y=0 \end{gathered}$

The consistent system has exactly one solution, it is independent .