Let it be x the numerator. Then we have:
• x + 3: Denominator.
,
• x + 9: Numerator increased by nine.
,
• (x + 3) + 9: Denominator increased by nine.
Since the simplified result of the fraction is 13/14, we can write and solve for x the following equation.
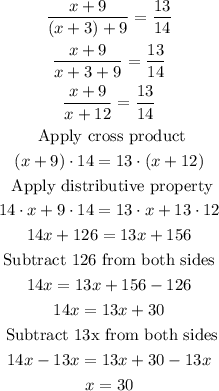
Now, we replace the value of x into the left expression from the equation we just solved.
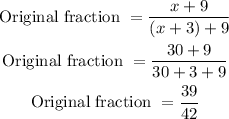
Therefore, the original fraction is 39/42.