The first thing we are going to do is identify the volume and surface of the cube and their respective derivatives or rate of change

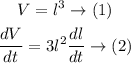

From the exercise we know that:
![\begin{gathered} (dV)/(dt)=300\frac{\operatorname{mm}^3}{s}\to(5) \\ 3l^2(dl)/(dt)=300\frac{\operatorname{mm}^3}{s}\to(2)=(5) \\ (dl)/(dt)=(300)/(3l^2)\frac{\operatorname{mm}^3}{s}\to(6) \end{gathered}]()
The exercise asks us to calculate the rate of change of the surface (4) so we substitute the differential of length (6) in (4)
![\begin{gathered} (dS)/(dt)=12\cdot l\cdot((300)/(3l^2)\frac{\operatorname{mm}^3}{s}) \\ (dS)/(dt)=(1200)/(l)\frac{\operatorname{mm}}{s} \end{gathered}]()
what is the rate of change of the cube’s surface area when its edges are 50 mm long?
![\begin{gathered} l=50\operatorname{mm} \\ (dS)/(dt)=\frac{1200}{50\operatorname{mm}}\frac{\operatorname{mm}^3}{s} \\ (dS)/(dt)=24\frac{\operatorname{mm}^2}{s} \end{gathered}]()
The answer is 44mm²/s