First we need to understand what |x| means or what values it repressents
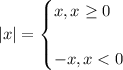
|x| indicates the absolute value of x, this is, x is always going to be positive, for example,
when x = 1 -> |x| = 1 , but also when x = -1 , then |x| = 1
Since, in this case, we need to find the limit when X approaches 0 from the left we are going to use |x| = -x , for x<0
this is...

At this point we have proved the limit statement.
So, in order to answer the question in the lower part... x approaches to 0 from the left, x<0, |x| = -x
In the graph you can see, whenever X<0 the value of the funcion will be negative and when it approaches 0 it becomes -1
On the other hand, when the function approaches to 0 from the right, the value of the function is +1. This is a discontinuity
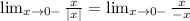
This way we eliminate the absolute value, because, remember, when x<0, |x| = -x