Answer:
95% of the data is represented as 69 to 93 (option G)
Step-by-step explanation:
Given:
mean of data = 81
standard deviation = 6
To find:
The option that represents 95% of the data
To determine the right option, we will apply the empirical rule (68-95-99.7%):
68% of the data will fall within 1 standard deviation
95% of the data will fall within 2 standard deviation
99.5% of the data will fall within 3 standard deviation
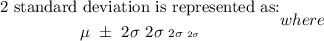
substitute the values:
