Given data:
The mass of ball 1 is m.
The mass of ball 2 is 1.26m.
The initial speed of ball 1 is u=5.4 m/s.
The final speed of the ball 1 U=2.6 m/s.
The angle at which the ball 1 moves from x-axis is θ=36.9.
Applying the conservation of momentum in x-direction,
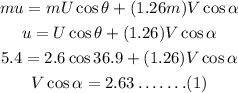
Here, V is the final speed of ball 2, and α is the angle of ball 2 with x-axis after the collision.
Applying the conservation of momentum in y-direction,

Dividing equation (2) and (1),

Subsitute the value of α in equation (1),
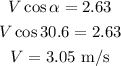
Thus, the final speed of the ball 2 (orange ball) is 3.05 m/s, and the direction of the orange ball is 30.6⁰.