the equation given was
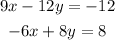
now to solve this equation, we should solve the simultaneous equation and get the values of x and y
now, let's take equation 1 and solve for x
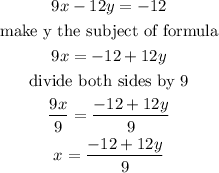
put x into equation 2
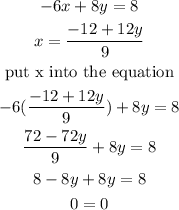
from the solution, y = 0
put y = 0 into either equation 1 or 2
from equation 1

from the above calculation, the above equation has only one solution.
the ordered pair is
