Question

Assume that adults have IQ scores that are normally distributed with a mean of 100 and a standard deviation of 15 (as on the Wechsler test). Find the probability that 2 - a randomly selected adult has an IQ between 100 and 120?

Answer 1

`\begin{equation*} 0.40824 \end{equation*}`

Step-by-step explanation

We want to find the probability that a randomly selected adult has an IQ between 100 and 120.

To do this, first, we have to find the z-score for 100 and 120 using the formula:

`z=(x-\mu)/(\sigma)`

where x = IQ score

σ = standard deviation

μ = mean

Hence, for an IQ score of 100, the z-score is:

`\begin{gathered} z=(100-100)/(15)=(0)/(15) \\ z=0 \end{gathered}`

For an IQ score of 120, the z-score is:

`\begin{gathered} z=(120-100)/(15)=(20)/(15) \\ z=1.33 \end{gathered}`

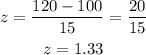
Now, to find the probability of an IQ score between 100 and 120, apply the formula:

Therefore, the probability is:

[tex]\begin{gathered} P(0That is the answer.