Question

Can you please help me out with a question

Answer 1

We have the following diagram

We are told that the arc NOL has an angle measure of 300°. Recall that the angle measure of the whole circle is 360°. Since the whole circle is the sum of the measures of arcs LMN and NOL we have that the measure of the arc LMN is

`\text{LMN+NOL=360}`
`\text{LMN}+300=360`

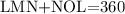
By subtracting 300 on both sides, we get

`\text{LMN=360-300=60}`

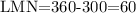
so arc LMN has a measure of 60°. However, note that measure of the arc LMN is the sum of the measures of arcs LM and MN. So

`LM+MN=\text{LMN}=60`

Now, note since lines MX and LM are perpendicular, we can do the following drawing

We can take a look at triangles LDX and NDX. Since the angles NDX and XDL are perpendicular, we can think of line MX as an axis of symmetry. That is, the left side of the circle with respect line MX is an exact copy of what is on the right. This means that the measure of the arc LM is the same as the measure of the arc MN. So we have that

`LM\text{ + MN = MN+MN=2MN=60}`

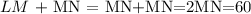
So, dividing both sides by 2, we get

`MN\text{ =}(60)/(2)=30`

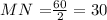
So the measure of the arc MN is 30°.