Question

Find the slope of each.1. (0, 4) and (2, -3)

Answer 1

slope = -3.5

Step-by-step explanation:

The points we have are:

`\begin{gathered} (0,4) \\ (2,-3) \end{gathered}`

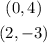
We have to label the coordinates as follows:

`\begin{gathered} x_1=0 \\ y_1=4 \\ x_2=2 \\ y_2=-3 \end{gathered}`

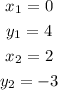
And now we use the formula to calculate the slope between the points:

`m=(y_2-y_1)/(x_2-x_1)`

We substitute the known values:

`m=(-3-4)/(2-0)`

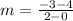
And solve the operations to find the slope "m" between the points:

`\begin{gathered} m=(-7)/(2) \\ m=-3.5 \end{gathered}`

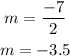
Answer: slope = -3.5