ANSWER:
5.32 m/s
Explanation:
We can determine the velocity from the following equation (initial kinetic energy:
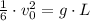
Where L is the length of the rod and g would be gravity, we replace and calculate the value of the initial velocity:
![\begin{gathered} v^2_0=6\cdot9.81\cdot0.48 \\ v^{}_0=\sqrt[]{28.2528} \\ v_0=5.32\text{ m/s} \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/hc0uasgcoa07z73m6w5snlb0qxwow53qdb.png)
The velocity is 5.32 m/s