Question

use the graph of y=-x/3 -1 determine which of the ordered pairs of the solution to the equation select all correct answers

Answer 1

Given:

`y=-(x)/(3)-1`

We have the graph below:

To determine the correct ordered pairs, let's solve for each of them.

a) (x, y) ==> (0, -1)

From the equation, substitute 0 for x and -1 for y:

`\begin{gathered} y=-(x)/(3)-1 \\ \\ -1=-(0)/(3)-1 \\ \\ -1=0-1 \\ \\ -1=-1 \\ \\ \text{Therefore (0, -1) is a solution} \end{gathered}`

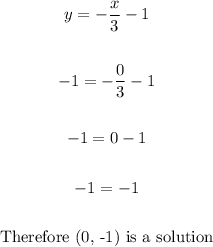
b) (x, y) ==> (3, -2)

Substitute 3 for x and -2 for y:

`\begin{gathered} y=-(x)/(3)-1 \\ \\ -2=-(3)/(3)-1 \\ \\ -2=-1-1 \\ \\ -2=-2 \\ \\ (3,\text{ -2) is a solution} \end{gathered}`

c) (x, y) ==> (3, -5)

Substitute 3 for x and -5 for y:

`\begin{gathered} y=-(x)/(3)-1 \\ \\ -5=-(3)/(3)-1 \\ \\ -5=-1-1 \\ \\ -5=-2 \\ \\ (3,\text{ -5) is not a solution} \end{gathered}`

d) (0, -5)

Substitute 0 for x and -5 for y:

`\begin{gathered} y=-(x)/(3)-1 \\ \\ -5=-(0)/(3)-1 \\ \\ -5=0-1 \\ \\ -5=-1 \\ \\ (0,\text{ -5) is not a solution} \end{gathered}`

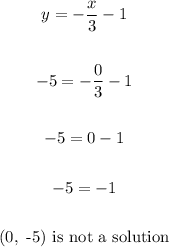
e) (x, y) ==> (-3, 0)

Substitute -3 for x and 0 for y:

`\begin{gathered} y=-(x)/(3)-1 \\ \\ 0=-(-3)/(3)-1 \\ \\ 0=1-1 \\ \\ 0=0 \\ \\ \text{The ordered pair (-3, 0) is a solution} \end{gathered}`

ANSWER:

(0, -1)

(3, -2)

(-3, 0)