Question

A) show the forces acting on the block in a labelled sketch when the surface is tilted. Treat the cylinder as a point.B) what is the maximum angle the rough surface can make with the horizontal before the cylinder topples over give answer to nearest degree

Answer 1

Given:

Diameter, d = 20 cm

Height, h = 40 cm

Mass, m = 10 kg

Given that the surface is slowly inclined to the horizontal, let's find the following:

• (A) Show the forces acting on the block in a labelled sketch when the surface is tilted. Treat the cylinder as a point.

The forces acting on the block are sketched below:

• (B) What is the maximum angle the rough surface can make with the horizontal before the cylinder topples over give answer to nearest degree.

The normal force (N) = 0 at the time the cylinder topples.

Before the the cylinder topples, tnet = 0

Apply the formula:

`mg\sin \theta\ast(h)/(2)-mg\cos \theta\ast(d)/(2)=0`

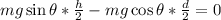
Rewrite the equation for θ:

`\begin{gathered} \sin \theta h=\cos \theta d \\ \\ (\sin\theta)/(\cos\theta)=(d)/(h) \\ \\ \tan \theta=(d)/(h) \\ \\ \theta=\tan ^(-1)((d)/(h)) \end{gathered}`

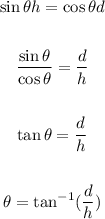
Where:

d = 20 cm

h = 40 cm

We have:

`\begin{gathered} \theta=\tan ^(-1)((20)/(40)) \\ \\ \theta=\tan ^(-1)(0.5) \\ \\ \theta=26.57\approx27^0 \end{gathered}`

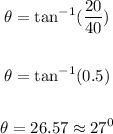
Therefore, the maximum angle the rough surface can make with the horizontal before the cylinder topples over is approximately 27 degrees.