The following image shows a diagram (not to scale) of the triangle with the indicated measurements:
We will label them as "a" and "b" for reference:
And we need to find the hypotenuse of the triangle, which is the side that is opposite to the 90° angle. We will label the hypotenuse as "c":
To solve the problem we have to us The Pythagorean Theorem:

Substituting the values of the legs a and b:

Since 60^2=3,600 and 80^2=6,400:
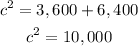
Finally, to find the hypotenuse "c", take the square root of both sides of the equation:
![\begin{gathered} \sqrt[]{c^2}=\sqrt[]{10,000} \\ c=\sqrt[]{10,000} \\ c=100 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/up4ji4q6oivb2vzkgepm1d2ox1bk8bo4g2.png)
The length of the hypotenuse is 100 cm.
Answer: 100cm