Question

Find the point-slope equation of the line using the point (7, 4) and slope of2Use the slash key (/) to indicate a fraction.

Answer 1

The general equation of a line is given as:

y = mx + c where

m = slope

c = intercept on y axis.

We are given a point (x,y) so we use the relation below to develop the equation.

`\begin{gathered} (y-y_1)/(x-x_1)=slope \\ \text{where:} \\ y_1=\text{ 4} \\ x_1=7 \end{gathered}`
`(y-4)/(x-7)=2`

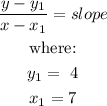
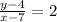
Crossmultiplying, we have:

2x - 14 = y - 4

Adding 4 to both sides,

y = 2x - 14 + 4

y = 2x - 10