Question

3.Find the values of x and y. Leave your answers in simplest radical form.6022yxX =y =Page

Answer 1

To calculate the values of x and y we need to apply the trigonometric relations to the right triangle. For this we will use the sine and cosine, their expressions are shown below:

`\begin{gathered} \sin (\alpha)=\frac{\text{ opposite cathetus}}{\text{ hypothenuse}} \\ \cos (\alpha)=\frac{\text{ adjacent cathetus}}{\text{ hypothenuse}} \end{gathered}`

Where alpha is the angle we know, the opposite cathetus is the cathetus that is farther from the angle and the adjacent cathetus is the cathetus that is closer to the angle. With this in mind we can use the data from the problem to calculate x and y.

`\begin{gathered} \sin (60)=(x)/(22) \\ 0.866=(x)/(22) \\ x=22\cdot0.866=19.052 \end{gathered}`
`\begin{gathered} \cos (60)=(y)/(22) \\ 0.5=(y)/(22) \\ y=0.5\cdot22=11 \end{gathered}`

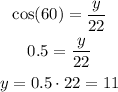
The value of x is equal to 19.052 and y is equal to 11.