Supposing the rate is:
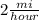
(a) Converting tho mi/day
Knowing that 1 day = 24 hours

The rate is 48 mi/day.
(b) Finding how many days will it take to reach 14 1/2
14 1/2 is the same as 14.5
Dividing 14.5 by 48:
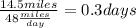
It will take 0.30 days.
(c) Finding when it will reach the destination.
Knowing the rate is 2 mi/hour, let's find how many hours it will take to reach 14.5 miles.
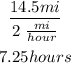
7.25 hours is 7 hours + 1/4 hour (15 min)
So, let's sum 06 (starting time) + 07 hours + 15 min
01:15 pm.
He will reach her destination at 01:15 pm.
Answer:
- (a) The rate is 48 mi/day.
- (b) It will take 0.30 days.
- (c) He will reach her destination at 01:15 pm.