Step 1
Given;
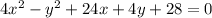
Required; To find the center that eliminates the linear terms
Step 2

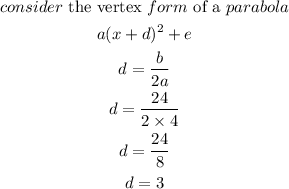
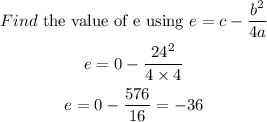
Step 3
Substitute a,d,e into the vertex form
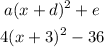

Step 4
Completing the square for -y²+4y

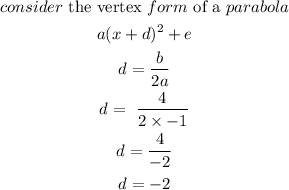
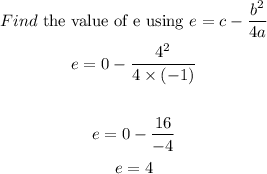
Step 5
Substitute a,d,e into the vertex form
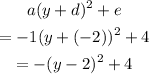
Step 6
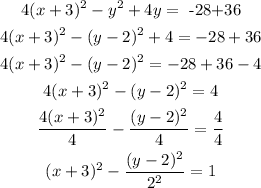
Step 7

Hence the answer is (-3,2)