Question

The length of a rectangle is 1m more than twice the width, the area of the rectangle is 45m^2

Answer 1

Let l and w be the length and width of the rectangle, respectively; therefore, according to the question

`\begin{gathered} l=2w+1 \\ and \\ l*w=45 \end{gathered}`

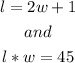
Where l and w are in meters.

Substitute the first equation into the second one, as shown below

`\begin{gathered} l=2w+1 \\ \Rightarrow(2w+1)*w=45 \\ \Rightarrow2w^2+w=45 \\ \Rightarrow2w^2+w-45=0 \end{gathered}`

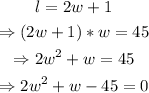
Solve for w using the quadratic formula,

`\begin{gathered} \Rightarrow w=(-1\pm√(1+4*2*45))/(2*2)=(-1\pm√(361))/(4)=(-1\pm19)/(4) \\ \Rightarrow w=(9)/(2),-5 \end{gathered}`

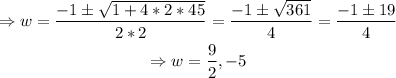
But w has to be positive since it is a measurement; therefore, w=9/2.

Finding l given the value of w=9/2,

`\begin{gathered} w=(9)/(2) \\ \Rightarrow l=2((9)/(2))+1=10 \\ \Rightarrow l=10 \end{gathered}`

Thus, the answers are length=10 m, width=4.5m