Question

How do i solve this problem? Hint: The following are the three relevant equations:a = G M / r2a = v2/rT = 2πr/vEliminating a and v and solving for r gives:r = 3√(GMT2/(4π2))Be sure to plug in the period in seconds. This will provide the orbital radius from the center of the earth. Using this value and the radius of the earth, we can determine the required height above the earth's surface. Finally, we can use v = 2πr/T to find the orbital speed.

Answer 1

An engineer wants a satellite to orbit the Earth with a period of 48 hours.

The acceleration due to gravity at the surface of the Earth is given by

`a=\frac{GM}{r^2^{}}`

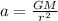
Where G is the gravitational constant (G = 6.67430×10⁻¹¹ Nm²/kg²), M is the mass of the Earth (m = 5.97x10²⁴ kg)

The acceleration of the satellite in a circular motion (centripetal acceleration) is given by

`a=(v^2)/(r)`

Where v is the speed of the satellite.

Equating both equations, we get

`\begin{gathered} (GM)/(r^2)=(v^2)/(r) \\ (GM)/(r)=v^2 \end{gathered}`

Substitute v = 2πr/T into the above equation

`\begin{gathered} (GM)/(r)=((2\pi r)/(T))^2 \\ (GM)/(r)=(4\pi^2r^2)/(T^2) \\ GM=(4\pi^2r^2\cdot r)/(T^2) \\ GM=(4\pi^2r^3)/(T^2) \\ r^3=(GMT^2)/(4\pi^2) \\ r=\sqrt[3]{(GMT^2)/(4\pi^2)} \end{gathered}`

So, we have got the equation for radius r.

Let us first convert the period from hours to seconds

`T=48*60*60=172800\; s`

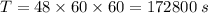
Substitute it into the above equation and find the radius (r).

`\begin{gathered} r=\sqrt[3]{(6.67430*10^(-11)\cdot5.97*10^(24)\cdot(172800)^2)/(4\pi^2)} \\ r=67045443\; m \\ r=6.7045443*10^7\; m \end{gathered}`

Therefore, the satellite must orbit at an orbital radius of 67,045,443 m

(b) How far is this above the Earth's surface?

The required height above the earth's surface can be found by subtracting the radius of the earth from the orbital radius from the center of the earth that we calculated in the previous part.

The radius of Earth is 6.37×10⁶ m

`\begin{gathered} h=6.7045443*10^7-6.37*10^6 \\ h=60675443\; m \\ h=6.0675443*10^7\; m \end{gathered}`

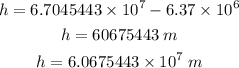
Therefore, the required height above the earth's surface is 60,675,443 m

(c) Speed of the satellite

The speed of the satellite is given by

`\begin{gathered} v=(2\pi r)/(T) \\ v=(2\cdot\pi\cdot67045443)/(172800) \\ v=2,437.84\; \; (m)/(s) \end{gathered}`

Therefore, the orbital speed of the satellite is 2,437.84 m/s