Question

Find the equation of the line containing the points (42.3,82) and (42.8,94) more

Answer 1

Let's remember that the equation of a line always has the form:

`y=m\cdot x+b`

where "m" and "b" are constant numbers that we must find. Now, let's find "m" first. "m" is called the slope of the line, and it represents the relationship between the changes in y (second component) and the changes in x (first component). So it isn't surprising that we can compute it by:

`m=(94-82)/(42.8-42.3)=(12)/(0.5)=24`

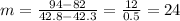
Having calculated "m", we know that, (for the point (42.3,82) must lie in the line)

`82=24\cdot(42.3)+b`

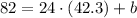
Then,

`b=82-24\cdot(42.3)=933.2`

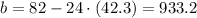
This implies that the equation of our line is

`y=24\cdot x-933.2`

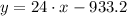
Here is a graph of the line:

Comment: Our line is represented with a red color.