For this problem we have a point given (5,y) and we know that this point is on a terminal side of an angle, we also know that:
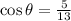
If we know the cos then we can find the sin on this way:
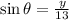
Then we can apply the following identity from trigonometry:
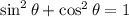
Using this formula we got:
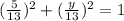
And we can solve for y:
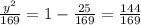
And solving for y we got:
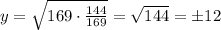
And the two possible solutions for this case are y=12 and y=-12