Question 1.
The free body diagrams of the situation are shown below:
The forces applied on m1 are in only the y direction then we only have one equation of motion to get it we use Newton's Second law. Then we have:

The forces applied on m2 are in the x and y direction, this means that we have two equations of motion. We don't expect this block to move on the y-direction (which we defined); this means that the acceleration in this direction has to be zero. Furthermore, since the blocks m1 and m2 are attach thorugh the rope their acceleration has to be the same; this means that the acceleration of block m2 in the x direction has to be a. Applying this and Newton's second law we have for block 2:
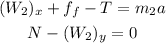
where (W2)x and (W2)y denote the component of the weight in the x and y direction, respectively. Now, since block m2 is on the verge of sliding this means that the system is in equilibrium, that is, the acceleration of the system is equal to zero. Then we have that the equations above take the form:
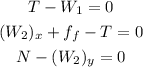
To determine the coefficient of static friction we firs need to determine the force of fricction. To do this we first solve the first equation for T:
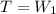
Now we plug this value in the second equation and solve for the force of friction:
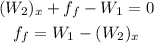
Now we need to remember that the force of friction can be obtain by:
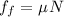
where mu is the coefficient of friction (this rule applies for static and kinetic friction). Then we have:

To find the normal force we use the third equation of motion:

Hence we have:
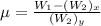
Now we need to remember that the components of the weight on an inclined plane are given by:

where theta is the angle of the plane.
Plugging this on the expression for the coefficient of friction:
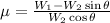
Finally we plug the values given to find the coefficient:
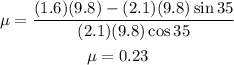
Therefore the coefficient of static friction is 0.23
Question 2
For this question we are going to look at the two block in the inclined plane as one block with mass of 4.2 kg and we are going to name the two blocks as m2.
The free body diagram in this case is:
Applying Newton's second law in each mass lead to the system of equations:
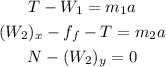
From the first equation of motion we have:

Plugging this in the second equation:
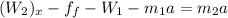
but we know that:
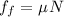
and:

Then we have:

Solving for the coefficient and using the expression for the wight on an inclined plane we have:
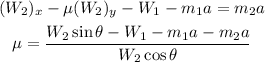
Plugging the values given we have:

Therefore the coefficient of kinetic friction is 0.18.
Question 3.
To find the coefficient of static friction between the two blocks on the inclined plane we need that the upper block to be stationary with respect to the lower block, that means that the friction has to be equal to the weight in the x-direction, that is:
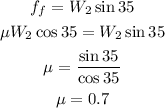
Therefore the coefficient between the blocks has to be at least 0.7