Notice that the problem is described by a right angle triangle for which we know the length of the hypotenuse (400 ft), and also know one of the triangle's acute angles (25 degrees)
Base on this knowledge, we can start by finding the other acute angle of the triangle (
This means : 25 + 90 +
Then we can solve for angle
115 +
subtract 115 degrees from both sides to isolate
< B = 180 - 115 = 65
then we have the measure of angle < B = 65 degrees.
Now we can find the value of the side adjacent to the angle 25 degrees by using the cosine trigonometric ratio:
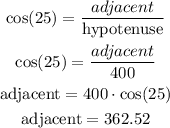
Then the side named "b" measures 362.52 ft
We can do something similar to find the measure of side a, but using the trigonometric ratio for the sine function:
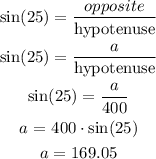
Then the measure of side a is 168.05 ft
Now, notice that the problem wants you to round the side measures to the nearest whole number, so you need to type the following:
a = 169 ft
b = 363 ft
angle