Question

A surveying crew has two points A and B marked along a roadside at a distance of 400 yd. A third point C ismarked at the back corner of a property along a perpendicular to the road at B. A straight path joining C to A forms a 28° angle with the road. Find the distance from the road to point C at the back of the property andthe distance from A to C using sine, cosine, and/or tangent. Round your answer to three decimal places.

Answer 1

In order to calculate the distance from B to C, we can use the tangent relation of the angle 28°.

The tangent is equal to the length of the opposite leg to the angle over the length of the adjacent leg to the angle.

So we have:

`\begin{gathered} \tan(28°)=(BC)/(AB)\\ \\ 0.5317094=(BC)/(400)\\ \\ BC=0.5317094\cdot400\\ \\ BC=212.684 \end{gathered}`

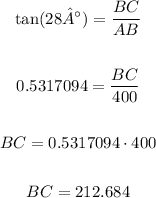
Now, to calculate the distance from A to C, we can use the cosine relation.

The cosine is equal to the length of the adjacent leg to the angle over the length of the hypotenuse.

So we have:

`\begin{gathered} \cos(28°)=(AB)/(AC)\\ \\ 0.8829476=(400)/(AC)\\ \\ AC=(400)/(0.8829476)\\ \\ AC=453.028 \end{gathered}`