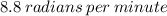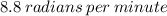
1) Gathering the data from the question:
Diameter = 200'
Spinning at 10mph
2) Let's convert the units to start working through that:

So, 1 mile=5280 ft and 1 hour = 60minutes. Then we can convert:

2.2) Since we have the diameter, then we can state the radius of this Ferris Wheel is 100 ft. Let's plug into the Circumference formula to get the circumference of the Ferris Wheel:
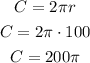
2.3) We can find the angular velocity since we have the speed and the Circumference. Note that the angular velocity is given as quotient between the speed and the circumference:
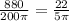
Note that this is given in revolutions per minute. And 1 revolution corresponds to one lap (2π radians). So we need another final conversion for the unit wanted for the question is radians per minute.
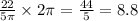
3) Thus the answer is: