The objective function is:
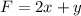
We need to find the shaded region where 4 inequalities overlap, then we need to graph the given inequalities:
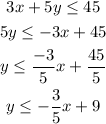
And the other one:
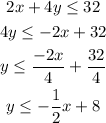
And x>=0, y>=0
The graph is:
The coordinates of the shaded region are then:
(0,0), (0,8), (10,3) and (15,0)
To obtain the maximum value, let's evaluate the objective function in all the coordinates:
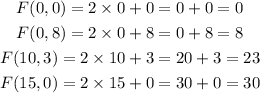
Then, the maximum value is the largest value obtained, then it's 30 and it occurs at x=15 and y=0.