Question

Inverse VariationThe variable y is inversely proportional to x if there is a nonzero constant, k, such that y=k/x.The number k is called the constant of variation or the constant of proportionality.Now, suppose that y is inversely proportional to x. If y is 2 when x is 7, we can find the constant of proportionality by first solving for k and then rewriting the equation to create an inverse variation equation.Start by substituting the values for y and x in to the standard equation and solve for k:2=k/714=ky=14/xPart AUse the equation we just found to determine the value of y when x = 21. Part BFind the value of x when y = 28. Part CLet’s look at a real-world example using inverse variation.In physics, Boyle’s law states that if the temperature is constant, then the pressure, P, of a gas is inversely proportional to the volume, V, of the gas. If the pressure of the gas in a cylinder is equal to 250 kilopascals when the volume of the container is 1.7 cubic meters, then determine the constant of proportionality for this situation. Show your work. Part DUse the constant of proportionality from part C to write an inverse variation equation to model this situation. Part EUsing the equation from part D, determine what the pressure would be in the container if the size of the container were to increase to 3.2 cubic meters. Part FWhat would the approximate volume need to be if you wanted the pressure to be 150 kilopascals? Part GReturning to the situation from part C, assume that the container was stored in a cooler room. Now, as the temperature of a gas increases, the pressure of the gas increases. Similarly, if the temperature of the gas decreases, the pressure of the gas decreases.So, assuming the container was placed in a cooler room, you know that the temperature of the gas has decreased by an unknown amount. Write an inequality to model this new situation. Part HUse the inequality from part G to write an inequality that represents the possible pressure of the gas if it is placed in a 3 cubic meter container. Give your answer in the form P < #. Part IYour answer from part H includes an infinite number of possibilities. However, in terms of this situation, some of the possible values are extraneous solutions. These are solutions that do not work given the situation. Rewrite your answer to remove any extraneous solutions and explain your answer.

Answer 1

Part A)

According to the text, the equation that relates x and y is:

`y=(14)/(x)`

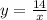
Substitute x=21 to find the value of y when x=21:

`y=(14)/(21)`

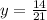
Simplify the expression:

`(14)/(21)=(7\cdot2)/(7\cdot3)=(2)/(3)`

Therefore, the value of y when x=21 is:

`(2)/(3)`

Part B)

To find the value of x when y=28, substitute y=28 and solve for x:

`\begin{gathered} y=(14)/(x) \\ \Rightarrow28=(14)/(x) \\ \Rightarrow28x=14 \\ \Rightarrow x=(14)/(28) \\ \therefore x=(1)/(2) \end{gathered}`

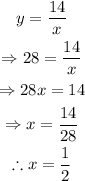
Therefore, the value of x when y=28 is:

`(1)/(2)`

Part C)

Since the pressure P is inversely proportional to the volume V, then:

`P=(k)/(V)`

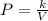
Solve for k and substitute P=250kPa and V=1.7m^2 to find the constant of proportionality:

`\begin{gathered} \Rightarrow k=PV \\ =(250\text{kPa})(1.7m^3) \\ =425\text{kPa}\cdot m^3 \end{gathered}`

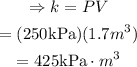
Therefore, the constant of proportionality for this situation is:

`425\text{ kPa}\cdot m^3`

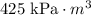
Part D)

Substitute the value of k into the equation that shows the inverse relation between P and V:

`\begin{gathered} P=(k)/(V) \\ \Rightarrow P=\frac{425\text{ kPa}\cdot m^3}{V} \end{gathered}`

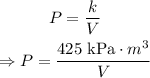
Therefore, the inverse variation equation model for this situation, is:

`P=\frac{425\text{ kPa}\cdot m^3}{V}`

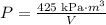
Part E)

Substitute V=3.2m^3 to find the pressure under those conditions:

`\begin{gathered} P=\frac{425\text{ kPa}\cdot m^3}{3.2m^3} \\ =132.8\text{kPa} \end{gathered}`

Therefore, the pressure would be:

`132.8\text{kPa}`

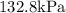
Part F)

Isolate V from the equation and substitute P=150kPa:

`\begin{gathered} V=\frac{425\text{ kPa}\cdot m^3}{P} \\ =\frac{425\text{ kPa}\cdot m^3}{150\text{ kPa}} \\ =2.83m^3 \end{gathered}`

Therefore, the approximate volume would have to be equal to:

`2.83m^3`

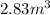
Part G)

Since the temperature has decreased, the pressure must be lower according to the description provided in the text. Then, an inequality to model this situation would be:

`P<(k)/(V)`

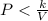
Part H)

Substitute the value of k and V=3m^3:

`\begin{gathered} P<\frac{425\text{ kPa}\cdot m^3}{3m^3} \\ \Rightarrow P<141.7\text{ kPa}^{} \end{gathered}`

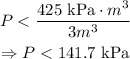
Part I)

Mathematically, all numbers under 141.7 satisfy the inequality from part H. Nevertheless, negative pressures do not have a physical meaning under the context of the Ideal Gas Law. Therefore, we must include the condition that P is greater than 0:

[tex]0