Let's draw a diagram of this problem:
This triangle can be seen as follows:
We can use the Law of Cosines to find the length of side c, since we know the measure of angle C:
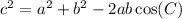
In our case:
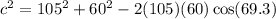
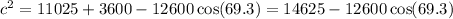
Taking the square root of both sides we get:
![c=\sqrt[]{14625-12600\cos (69.3)}](https://img.qammunity.org/2023/formulas/mathematics/high-school/k621z8cq4bajxv9iti8krmc4vlpvw7dgq5.png)
which, using a calculator or online resource to calculate the right side of the equation will give us:
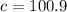
To the nearest yard, A and B are 101 yards apart, so option A. is correct.