Given:
the plan of the pre-paid cell phone
The plan has a monthly fee and a charge for each minute
Let the monthly cost = C
and the number of minutes = x
the general equation will be:
C = ax + b
Where (b) is the monthly fee, and (a) is the charge per minute
We will find the values of (a) and (b) using the following:
1) 430 minutes cost $227.50
2) 780 minutes cost $385.00.
So, we have the following equations:
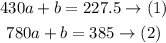
Solve the equations, subtract equation (1) from (2) to eliminate (b), and solve for (a):
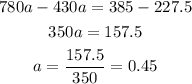
Substitute with (a) into equation (1) to find the value of (b)

So, the equation of the monthly cost will be:
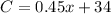
Part (b): When x = 484 minutes, we will find C
so, substitute with (x) into the equation of C

So, the answer will be:
A) C = 0.45x + 34
B) $251.8