In general, given a function g(x), a vertical stretch/compression is given by the transformation below
![\begin{gathered} g(x)\rightarrow a*g(x) \\ a>1\rightarrow\text{ stretch} \\ 0Therefore, in our case,[tex]x^2\rightarrow2x^2\Rightarrow\text{ vertical stretch by a factor of 2}]()
On the other hand, a vertical shift is given by the following transformation
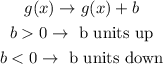
Thus,
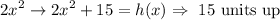
Hence, the answer is option C. Vertical stretch by a factor of 2 and a vertical shift by 15 units up.