To solve the question, we will have to determine the quadrant within which the point falls
Since both values are negative
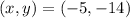
So the values are in the third quadrant
So we will have to get the magnitude first
![\begin{gathered} \text{Magnitude}=\sqrt[]{x^2+y^2} \\ \text{Magnitude}=\sqrt[]{(-5)^2+(-14)^2} \\ \text{Magnitude}=\sqrt[]{25+196} \\ \text{Magnitude}=221 \\ \text{Magnitude}=14.866 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/srwmq4l8ad3aljzz0xz89kynip6fecha2u.png)
Next, we will have to get the direction
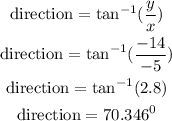
So, since we know that the point is on the third quadrant, then
We will add 180 degrees (90 degrees from first and 90 degrees from the second quadrant)
So we will have
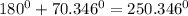
Thus the answer is
