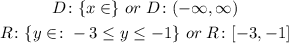Using
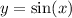
5 units right: Let's use the following rule:
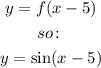
Reflect on x-axis: Let's use the following rule:
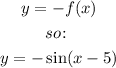
2 units down: Let's use the following rule:
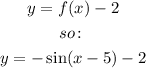
Horizontal compression with factor 2: Let's use the following rule:
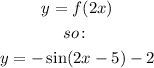
Let's graph the parent function, and the new function:
The blue graph is the parent function and the red graph is the new function after the transformations applied.
The domain and the range of the new function are: