Question

A solenoid consists of 4200 turns of copper wire. The wire has a diameter of 0.200 mm. The solenoid has a diameter of 1.00 cm. When the solenoid is connected to a 12.0 V battery, we observe that the current increases over time and is 155 mA after 1.50 milliseconds have passed. Assume that the internal resistance of the battery and connecting wires is negligible.

Required:
a. What is the length of wire needed to form the solenoid?
b. What is the inductance of the solenoid?
c. What is the length of the solenoid?
d. What will be the current after three time constants have elapsed?

Answer 1

a. The length of the solenoid wire is approximately 131.95 m

b. The inductance of the solenoid is approximately 2.078 × 10⁻³ H

c. The length of the solenoid is 0.84 m

d. The current after three time constants have elapsed is approximately 456.1 A

Step-by-step explanation:

The given parameters are;

The number of turns in the solenoid, N = 4,200 turns

The diameter of the wire, d = 0.200 mm

The diameter of the solenoid, D = 1.00 cm

The voltage of the battery connected to the solenoid, V = 12.0 V

The current increase = 155 mA

The time for the increase = 1.50 millisecond

The internal resistance of the battery is negligible

a. The length of wire needed to form the solenoid, l = π·D·N

∴ l = π × 0.01 × 4,200 ≈ 131.95

The length of the solenoid, l ≈ 131.95 m

b. The inductance, 'L', of the solenoid is given as follows;

`L = (\mu_0 \cdot N^2 \cdot A)/(l)`

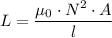
Where;

μ₀ = 12.6 × 10⁻⁷ H/m

N² = 4,200²

A = The cross sectional area of the solenoid = π·D²/4

l = Length of the solenoid = d × N = 0.0002 m × 4,200 = 0.84 m

∴ L = (12.6 × 10⁻⁷ × 4,200² × 0.01² × π/4)/0.84 ≈ 0.002078 = 2.078 × 10⁻³

The inductance, L ≈ 2.078 × 10⁻³ H

c.) The length of the solenoid = d × N = 0.0002 m × 4,200 = 0.84 m

The length of the solenoid = 0.84 m

d. The current after three time constant

We have;

∈ = -L × di/dt

di/dt = 155 mA/1.5 ms = 103.
`\overline 3` A/s

∈ = 103.
`\overline 3` A/s × 2.078 × 10⁻³ H = 0.21472
`\overline 6` V

We have;

`\tau = (t)/(\left(ln(1)/(1-(Change)/(Final-Start) ) \right))`

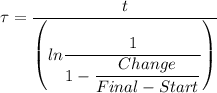
The change in voltage = 0.21472
`\overline 6` V

The start voltage = 0 V

The final voltage = 12.0 V

t = 1.5 ms = 0.0015 s

We get;

`\tau = (0.0015)/(\left(ln(1)/(1-(0.21472\overline 6)/(12-0) ) \right)) \approx 8.3076* 10^(-2)`

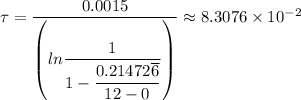
τ = L/R

Therefore,

R = L/τ =

The resistance = 2.078 × 10⁻³/(8.3076×10⁻²) = 0.0250

The resistance = 0.0250 Ω

`I= (V)/(R) \cdot \left(1 - e^{-(t)/(\tau) }\right)`

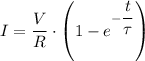
Therefore, after three time constants, we have;

∴ I = (12.0/(0.0250)) × (1 - e⁻³) ≈ 456.1

The current after three time constants have elapsed, I ≈ 456.1 A.