Question

Find the equation for the line that passes through the point (-4,-3) and that is perpendicular to the line with the equation y=3/4x-1

Answer 1

Given,

The coordinate that lie on the line is (-4, -3).

The equation of line is y = 3/4x-1.

The standard equation of line is,

`y=mx+c`

Here, m is the slope of the line.

On comparing, the slope of the line y = 3/4x-1 with the standard equation of line then m = 3/4.

The relation of two perpendicular line is,

`\begin{gathered} m_1* m_2=-1_{} \\ (3)/(4)* m_2=-1 \\ m_2=(-4)/(3) \end{gathered}`

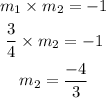
The equation of line passing through the point (-4,-3) and perpendicular to line y = 3/4x-1 is,

`\begin{gathered} y-y_1=m(x-x_1) \\ y-(-3)=(-4)/(3)(x-(-4)) \\ y+3=(-4)/(3)(x+4) \\ 3y+9=-4x-16 \\ 3y=-4x-25 \\ y=(-4x-25)/(3) \end{gathered}`

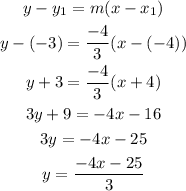
Hence, the equation of line perpendicular to y = 3/4x-1 is y = (-4x-25)/3.