The slope of a line is given by the following formula:

Where (x1, y1) and (x2, y2) are the coordinates of two points where the line passes through. By replacing (2, -9) and (8, -6) into the above equation, we get:
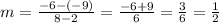
Then, the slope of the given line is 1/2. The equation of a line can be written in slope-intercept form like this:
y = mx + b = (1/2)x + b
We can find the value of b by replacing the coordinates of one of the point where the lie goes through, let's take (2, -9), then we get:
-9 = (1/2)(2) + b
-9 = 1 + b
-9 - 1 = 1 - 1 + b
-10 = b
b = -10
Then, we can rewrite the above equation to get: y = (1/2)x - 10