Question

Use the circle unit to evaluate csc(-/2)

Answer 1

The definition of the cosecant function is

`\csc \theta=(1)/(\sin \theta)`

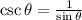
Therefore,

`\Rightarrow\csc (-(\pi)/(2))=(1)/(\sin (-(\pi)/(2)))`

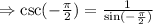
To find sin(-pi/2), use the diagram below.

Consider that the circumference has a radius equal to 1. Then, the coordinates of the orange point are (0,-1). Furthermore, the points on the circumference are given as (cos(theta), sin(theta)); therefore,

`\begin{gathered} \Rightarrow(0,-1)=(\cos (-(\pi)/(2)),\sin (-(\pi)/(2))) \\ \Rightarrow\sin (-(\pi)/(2))=-1 \\ \Rightarrow\csc (-(\pi)/(2))=(1)/(-1)=-1 \\ \Rightarrow\csc (-(\pi)/(2))=-1 \end{gathered}`

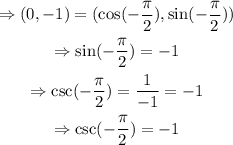
Thus, the answer is csc(-pi/2)=-1