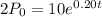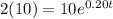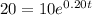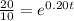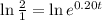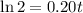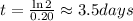Question

The population of a culture of bacteria, P(t), where t is time in days, is growing at a rate that is proportional to the population itself and the growth rate is 0.2. The initial population is 10.

Answer 1

Step-by-step explanation

Using the formula for the population growth:

`P(t)=P_0\cdot(1+r)^t`

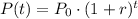
where P₀ is the initial population, r is the rate of growth, and t is the time.

From the given information, we know that:

• P₀ = 10

,

• r = 0.2

1.

And we are asked to find P(50) (when t = 50), thus, by replacing the values we get:

`P(50)=10\cdot(1+0.20)^(50)`
`P(50)\approx91004.3815`

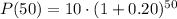
2.

For the population to double, this would mean that P(t) = 2P₀. By replacing this we get:

`2P_0=10e^(0.20t)`
`2(10)=10e^(0.20t)`
`20=10e^(0.20t)`
`(20)/(10)=e^(0.20t)`
`\ln(2)/(1)=\ln e^(0.20t)`
`\ln2=0.20t`
`t=(\ln2)/(0.20)\approx3.5days`