1 Answer

Thiagoveloso · Answer 1 · 2023-05-01T01:35:56+0000

We have that the measurement of three angles of a triangle is:

1. Angle 1: 2x degrees.

2. Angle 2: 3x degrees.

3. Angle 3: (x+30) degrees.

We know that the sum of the internal angles of a triangle is equal to 180.

Therefore, to find the value of x, we can proceed as follows:

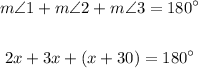
$\begin{gathered} m\angle1+m\angle2+m\angle3=180^(\circ) \\ \\ 2x+3x+(x+30)=180^(\circ) \end{gathered}$

Now, we can add the like terms as follows:

$\begin{gathered} 2x+3x+x+30^(\circ)=180^(\circ) \\ \\ 5x+x+30^(\circ)=180^(\circ) \\ \\ 6x+30^^(\circ)=180^(\circ) \end{gathered}$

We can subtract 30 degrees to both sides of the equation, and then we have to divide both sides by 6:

$\begin{gathered} 6x+30^(\circ)-30^(\circ)=180^(\circ)-30^(\circ) \\ \\ 6x=150^^(\circ) \\ \\ (6x)/(6)=(150)/(6) \\ \\ x=25 \end{gathered}$

Therefore, in summary, the value for x is equal to 25.

Please log in or register to add a comment.