Answer:
7.) The coordinates of point K are (10,8)
8.) The length of the radius, to the nearest hundreth, is of 4.24 units.
Explanation:
P is the center of a circle with diameter KR:
This means that P is the midpoint between K and R.
I will see that the points are:
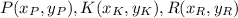
Since P is the midpoint:
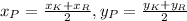
P(7,-5) and R(4,-2)
This means that:
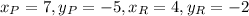
7. If P(7,-5) and R(4,-2), find the coordinates of point K

Substituting:
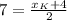
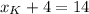
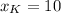
Now we do the same for y:
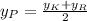
Replacing with what we have
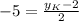
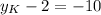
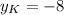
The coordinates of point K are (10,8)
8. What is the length of the radius to the nearest hundredth?
The radius is half the diameter(distance between KR divided by 2). It can also be the distance between an endpoint of the circle and the centre(Distance between P and K, or between P and R).
I am going to calculate the distance between P and R.
P(7,-5) and R(4,-2). So
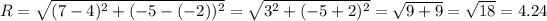
The length of the radius, to the nearest hundreth, is of 4.24 units.